Leonardo Fibonacci, a medieval mathematician, noticed a particular order or sequence that life utilizes to grow and discovered that this particular ratio kept coming up everywhere. Within the sequence, each number is the the sum of the previous two numbers. The first twenty Fibonacci numbers are:
1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, 610, 987, 1507, 1584, 4181, 6765, etc.
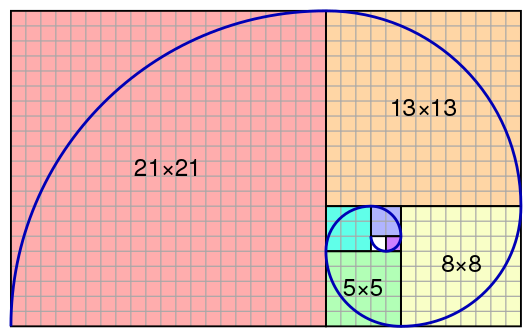
Originating in the golden mean spiral, this pattern shows up consistently in life, going in and out forever, without beginning or end. Life, which begins from a void, must begin somewhere from nowhere. Fibonacci's sequence is the answer.
If you divide one term of this sequence into the next one, and by continuing this process and calculating the average, you will find the number 1.6180339, also known as the phi ratio or golden mean. For example:
If you divide one term of this sequence into the next one, and by continuing this process and calculating the average, you will find the number 1.6180339, also known as the phi ratio or golden mean. For example:
1 divided by 1 = 1
2 divided by 1 = 2
3 divided by 2 = 1.5
5 divided by 3 = 1.66
8 divided by 5 = 1.6
13 divided by 8 = 1.625
21 divided by 13 = 1.615
34 divided by 21 = 1.619
55 divided by 34 = 1.617
89 divided by 55 = 1.6181
2 divided by 1 = 2
3 divided by 2 = 1.5
5 divided by 3 = 1.66
8 divided by 5 = 1.6
13 divided by 8 = 1.625
21 divided by 13 = 1.615
34 divided by 21 = 1.619
55 divided by 34 = 1.617
89 divided by 55 = 1.6181
From this list, the resulting numbers keep getting closer and closer to phi ratio, so close that you cannot tell the difference, though without ever actually attaining it. The Fibonacci spiral hows how the pattern work geometrically.
Image by Jahobr on Wikimedia Commons
References
Rose, N. (2014). The Golden Mean and Fibonacci Numbers
Scott, T. & Marketos, P. (2014) On the origin of the Fibonacci Sequence
Scott, T. & Marketos, P. (2014) On the origin of the Fibonacci Sequence